Marine piling - energy conversion factors in underwater radiated sound: review

4. Options For Sound Predictions

We have seen from previous sections that predicting radiated sound fields resulting from piling operations is not a straightforward task. There are, however, methods developed and being developed to further improve the sound level forecasts. These vary in complexity and in their range of applications with one comprehensive overview being provided by Tsouvalas (2020). A few approaches for sound level predictions are discussed further here.

4.1. Predictions from Existing Measurements

Due to the complexities in modelling sound levels from piling operations, there have been efforts directed into making simpler predictions possible whilst retaining a reasonable level of accuracy. One such effort involves the idea of using scaling factors. The general idea is that, if one has measurements of a piling operation, one can then use these measurements along with calculated adjustments to predict sound fields for the new scenario. The calculated adjustments arise from inputs including a change in the hammer, a change in the pile, or a change in the environment. Consequently, the value of the ECF, as considered in this report, essentially acts as the culmination of these scaling factors that affect the sound levels in the modelled domain.

There has been previous discussion regarding the use of 1.0 % or 0.5 % as an energy conversion factor. As shown in Section 2.1.2, however, calculated values of the ECF vary considerably. It should also be noted that calculations of the point-source equivalent ECF would have been performed using point source models which, as shown in Section 3, are strongly dependent on the measurement location and highly likely to yield an incorrect answer.

It is stressed that the concept of using the ECF is not flawed in itself. However, a single off-the-shelf value cannot capture the separate influences of the hammer properties, the pile properties, and the environment insomuch as how they affect the source level. If an energy conversion approach is to be used, it should be known how the different parameters affect the ECF, and by how much.

4.1.1. Scaling laws for unmitigated pile driving

The study, “Scaling laws for unmitigated pile driving: Dependence of underwater noise on strike energy, pile diameter. ram weight, and water depth” by von Pein et al. (2022) involved analysing the effect of changes in the mentioned aspects and the effect each had on the radiated noise. These parameters, the authors believed, would be the main influencers over the radiated noise.

The authors identify the limits of the method and recommend that they are most suitable for distances close to the pile stating that beyond 5 km the influence of frequency-dependent losses would require further attention. The effect of sediment changes was not investigated due to not being able to generalise soil effects in a simple scaling law. The analyses presented consider the sediment to be an infinite half-space, and as such any sub-bottom reflections and upward refraction effects are not considered. Finally, the study investigated only unmitigated piling operations at this stage.

In terms of hammer energy, it was found that assuming a linear dependence of acoustical energy on the strike energy agrees well with modelled results and reasonably well with measurements. This is in accordance with Figure 1, and also represented in Equation 1 presuming that β is independent of the hammer energy.

The effect of the pile diameter has been investigated previously with research by Nehls et al. (2007) suggesting that for a given blow energy, pile diameter alone does not necessarily lead to a change in noise output. The reasoning was that whilst there exists a larger surface area, for a constant energy, the amplitude would reduce. The von Pein report also highlights the ‘interplay’ of numerous factors affected by a change on the diameter on the radiated sound. For a rod, the radial expansion increases linearly with the radius presuming the stress remains the same. To maintain a constant stress in the larger diameter pile, however, the energy must also increase.

One important aspect of the diameter increase is a reduction in the pile ring frequency, f_r. It has been shown that if one considers that frequency content of the input energy from the hammer, energy between 0.5 f_r and 0.8 f_r is amplified and more efficiently converted into sound pressure waves than that above the ring frequency (Tsouvalas 2020). Consequently, the excitation force should be considered when determining the effect of a change in pile diameter.

The influence of the ram mass is that it directly affects the initial hammer velocity. The impact velocity, v₀, of the ram is v₀ = √2E_k/m_r where E_k is the kinetic energy, and m_r is the ram mass. Consequently, for a fixed hammer energy, an increase in ram mass results in a reduction of impact velocity. The reduction in impact velocity in turn leads to a reduction in the radiated sound. It is noted that the analysis presented in the paper excludes possible influences from changes in hammer element stiffnesses or dimensions, as these factors cannot be easily generalised.

Finally, the effect of a change in water depth is investigated based on transmission losses calculated using the DCS model. The result is that greater water depths result in fewer bottom reflections over a given distance, and thus produces increased sound levels.

It was found that using results from impact piling at 21 wind farms, the SEL at 750 m could be predicted to within -2.3 to +4.2 dB based on the scaling law formulations generated in the project. Deviations were believed to be caused by sediment parameters, interplay between the hammer and monopile combinations, weather and measurement conditions, and possible coupling effect, e.g., with jacket structures.

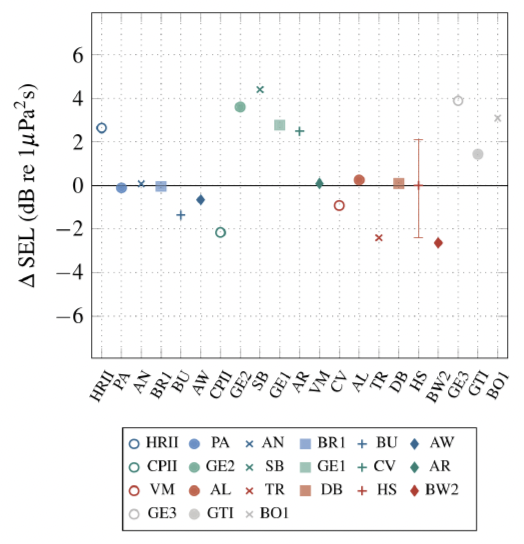
In conclusion, the authors recommend that scaling should either be undertaken over a small number of very similar piling situations or averaged over a large data set. Remaining errors are likely to be down to multiple hammer, pile, and environmental parameters that do not reduce simply to a single scaling factor. The aim of the project, however, was to develop a general tool to allow the calculation of general trends and first estimations.

4.1.2. Parametric Analysis and Sensitivity Study

Another approach to pile predictions is provided by the report “A Parametric Analysis and Sensitivity Study of the Acoustic Propagation for Renewable Energy” by Heaney et al. (2020). For this project, the aims were to investigate the sensitivity of the sound levels from piling based on numerous factors, and to provide guidance for future projects as to whether sound levels from new piling operations could be reasonably predicted based an existing set of results or whether results should be generated from new model runs.

Two sites off the United States Atlantic coast were selected for the sensitivity study: Rhode Island and Virginia Beach. The study considered a fixed pile size and hammer, and investigated how changes in the environment affected the radiated sound. The biggest effect on the sound levels was the selection of site, with site selection being a proxy not only for water depth at the source, but bathymetry across the surrounding region. For this, the differences in SEL were up to 40 dB beyond 20 km from the sites. The next largest influence on radiated sound was the sediment type; as harder sediments reflected more of the energy back into the water column, the sound propagated further than for softer sediment environments. Finally, it was found that the sound speed profile (SSP) had the smallest effect, with better propagation in the winter than in the summer; the warming of the sea surface in summer led to increased refraction towards the seafloor, resulting in more bottom bounces and greater losses.

The authors highlight that there are limiting factors to the use of the results of the study. Firstly, only a single type of pile and input energy was used across the study; the observation is that for different sizes of piles that the noise field will be different and would require new measurements or modelling. Secondly, only vertical piles were considered in this study, and that the sound field from raked piles would have strong azimuthal dependence.

The report recommendations regarding whether existing results could be applied to new results are summarised below:

• Sites that differ in water depth by 50 % should be modelled separately (e.g., water depths of 5, 7, 11, 16, 23, 34, 50 m); results can be interpolated between depths where available.
• Sites where the mean grain size differs by 1 φ or more should be modelled separately.
• Sites run for a different target month should be modelled separately to adequately capture the most likely SSP.
• Sites that differ in distance from the shore by 15 km should be modelled separately.
• Sites that use a different hammer or pile should be modelled separately.

4.2. Numerical Modelling

Numerical modelling allows for predictions of the piling noise with reasonable accuracy proving the model inputs and the method of propagation are appropriate. There are numerous advantages to using numerical modelling over the scaling approach in the previous section. There is no requirement for a reference sound level to be used, allowing forecasts to take place in new environments using new pile geometries and hammers. The use of numerical modelling allows for greater control over environmental inputs such as range-varying bathymetry and layered sediments. Additionally, the models are able to take the subterranean sound into account, are effective for propagation into deeper water, and can generate predictions of the sound levels as it varies with depth as well as range.

On top of these benefits, there are additional extended capabilities that can be employed in the modelling to take other situations into account. For example, in the case of underwater piling, more energy emanates from the embedded portion of the pile towards the latter stages of the operation which is harder to reproduce in the scaling or geometric models. Another factor is the use of mitigation strategies to reduce the acoustic impact of larger piles and larger hammers, including pile sleeves and bubble curtains. There are methods developed for numerical models to include such mitigation techniques that are currently beyond the capabilities of the simpler techniques.

Given the nature of piling, the description of the sediment is crucial in both the propagation and source function models for pile driving. In many numerical models, a linearised fluid sediment approximation is used. More recent models, however, have placed greater emphasis on incorporating elastic sediment properties to enhance the accuracy of calculating interface waves and the propagating field within the sediment (Tsouvalas 2020).

The modelling approaches used in numerical methods typically feature a close-range model coupled to a long-range model. The close-range model calculates the acoustic field very close to the pile having taken the pile stress wave into account, while the long-range acoustic model is typically optimised for acoustic propagation.

Numerical modelling of the pile itself is often done using finite-element models, although finite-difference models and semi-analytical models have been used successfully. The purpose of this module is to model the response of the pile to the input force (often derived from separate driveability models, or analytical functions), and how the pile response is manifest in the acoustic wave at the model boundary. This takes into account the progression of the stress wave down the pile with its reflections and the resulting conical wavefront emanating from the pile. The output of the close-range model is used as the input to the long-range model. Common options for the long-range model are described briefly in Section 2.2.1. Whilst there may have been more recent additions, Tsouvalas (2020) provides a list of those impact piling source and propagation models either validated against experimental data or numerical benchmark studies.